Your Star edge coloring of graphs images are ready. Star edge coloring of graphs are a topic that is being searched for and liked by netizens now. You can Find and Download the Star edge coloring of graphs files here. Find and Download all royalty-free photos.
If you’re searching for star edge coloring of graphs pictures information related to the star edge coloring of graphs interest, you have come to the ideal site. Our site frequently gives you hints for viewing the maximum quality video and image content, please kindly search and find more enlightening video articles and graphics that match your interests.
Star Edge Coloring Of Graphs. In this article we establish tight upper bounds for trees and subcubic outerplanar graphs and derive an upper bound for outerplanar graphs. That is at least three colors occur in a path and cycle of length four. The star chromatic index χ st G of a graph G is the smallest integer k for which G has a proper k -edge-coloring without bichromatic paths or cycles of length four. For Better Performance Please Use Chrome or Firefox Web Browser.
An Integer Linear Programming Approach To Graph Coloring Cu Denver Optimization Student Wiki From math.ucdenver.edu
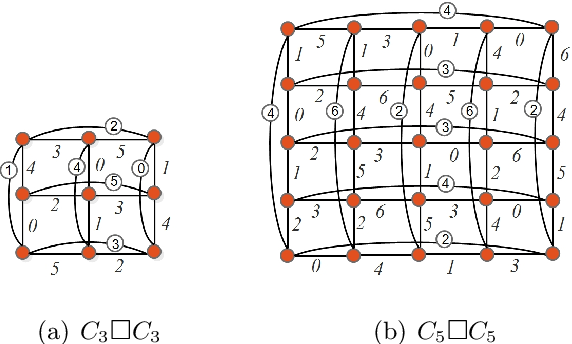
A proper edge coloring of a graph G is called star-edge coloring if there do not exist bichromatic paths and cycles of length four. The star chromatic index χstG of a graph G is the smallest. A star edge coloring of a graph G is a proper edge coloring of G such that no path or cycle of length 4 is bicolored. A star edge coloring of a graph is a proper edge coloring without bichromatic paths and cycles of length four. Star edge-coloring of Halin graphs. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively.
A star edge coloring of a graph G is a proper edge coloring of G such that no path or cycle of length 4 is bicolored.
That is de ned as follows. A proper edge coloring of a graph G is called star-edge coloring if there do not exist bichromatic paths and cycles of length four. For a graph G let the list star chromatic index of G ch. A star edge-coloring of a graph G is a proper edge coloring such that every 2-colored connected subgraph of G is a path of length at most 3. That is de ned as follows. The star chromatic index χ st G of a graph G is the smallest integer k for which G has a proper k -edge-coloring without bichromatic paths or cycles of length four.
Source: semanticscholar.org
In this paper we prove that 1 if G is a graph with Δ 4 then χ st G 14. The list star chromatic index chstG is defined. The star chromatic index of a graph G is the smallest integer k for which G admits a star edge coloring with k colors. The star chromatic number s G of Gis the least number of. In this paper we prove that 1 if G is a graph with Δ 4 then χ st G 14.
Source: researchgate.net
This bound is tight. In this paper we prove that 1 if G is a graph with Δ 4 then χ st G 14. The smallest integer kfor which Gadmits a k-star edge coloring is called the star chromatic index of Gand is denoted by 0 s G. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively. This bound is tight.
Source: wikiwand.com
In this paper we prove that 1 if G is a graph with Δ 4 then χ st G 14. Star edge-coloring of Halin graphs. The star chromatic index of a graph is the smallest integer for which has a proper -edge coloring without bichromatic paths or cycles of length four. The star chromatic number s G of Gis the least number of. The star chromatic index chi_ st G of a graph G is the smallest integer k for which G has a proper k-edge.
Source: mathworld.wolfram.com
Star edge-coloring of Halin graphs. Integer k such that G has a star k-edge-coloring. And 2 if G is a bipartite graph with. A star edge coloring of a graph G is a proper edge coloring of G such that no path or cycle of length 4 is bicolored. The star chromatic index chi_ st G of a graph G is the smallest integer k for which G has a proper k-edge.
Source: researchgate.net
A star edge coloring of a graph is a proper edge coloring without bichromatic paths and cycles of length four. This is called a vertex coloring. We call a star edge coloring of Gwith kcolors a k-star edge coloring of G. That is at least three colors occur in a path and cycle of length four. A star edge coloring of a graph G is a proper edge coloring of G such that every path and cycle of length four in G uses at least three different colors.
Source: link.springer.com
A star edge coloring of a graph is a proper edge coloring without bichromatic paths and cycles of length four. Star edge-coloring of Halin graphs. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively. Integer k such that G has a star k-edge-coloring. This bound is tight.
Source: researchgate.net
We call a star edge coloring of Gwith kcolors a k-star edge coloring of G. The star chromatic number s G of Gis the least number of. St G be the minimum k such that for any k-uniform list assignment L for the set of edges G has a star edge-coloring from L. And 2 if G is a bipartite graph with. In graph theory graph coloring is a special case of graph labeling.
Source: link.springer.com
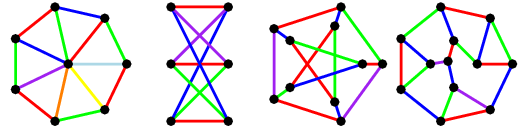
The star chromatic number s G of Gis the least number of. Equivalently in a star coloring the induced subgraphs formed by the vertices of any two colors has connected components that are star graphs. A star edge coloring of a graph G is a proper edge coloring of G such that every path and cycle of length four in G uses at least three different colors. It is an assignment of labels traditionally called colors to elements of a graph subject to certain constraints. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively.
Source: researchgate.net
Dvořák Mohar and. A star edge coloring of a graph is a proper edge coloring without bichromatic paths and cycles of length four. In graph theory graph coloring is a special case of graph labeling. Integer k such that G has a star k-edge-coloring. A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four.
Source: link.springer.com
A star k-edge-coloring is a proper k-edge-coloring such that every connected bicolored sub-graph is a path of length at most 3. A star edge coloring of a graph G is a proper edge coloring of G such that every path and cycle of length four in G uses at least three different colors. A star edge coloring of a graph G is a proper edge coloring such that every connected bicolored subgraph is a path of length at most 3 the length of a path is the number of edges. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively. Integer k such that G has a star k-edge-coloring.
Source: mathworld.wolfram.com
It is an assignment of labels traditionally called colors to elements of a graph subject to certain constraints. That is at least three colors occur in a path and cycle of length four. Star edge-coloring of Halin graphs. Equivalently in a star coloring the induced subgraphs formed by the vertices of any two colors has connected components that are star graphs. Professor of Mathematical Sciences.
Source: wikiwand.com
The star chromatic index of a graph G is the smallest integer k for which G admits a star edge coloring with k colors. We call a star edge coloring of Gwith kcolors a k-star edge coloring of G. A star edge coloring of a graph is a proper edge coloring without bichromatic paths and cycles of length four. For Better Performance Please Use Chrome or Firefox Web Browser. A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four.
Source: gatevidyalay.com
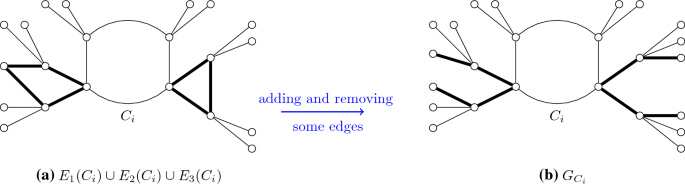
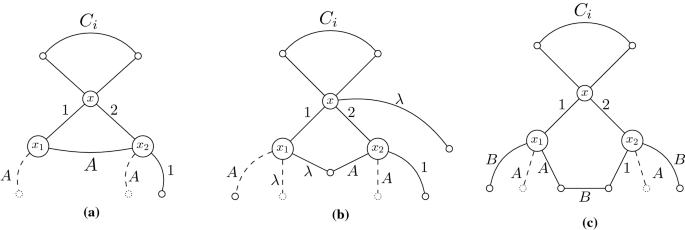
In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by P m C n P m W n P m H n P m G n respectively. A proper edge coloring of a graph G is called star-edge coloring if there do not exist bichromatic paths and cycles of length four. The star chromatic index of G denoted by chi prime _sG is the minimum k such that G admits a star edge coloring with k colors. A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four. In graph theory graph coloring is a special case of graph labeling.
Source: math.ucdenver.edu
Just like relation between concepts of traditional edge and vertex colorings a star coloring of a line graph is a star edge coloring of the original graph. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively. Integer k such that G has a star k-edge-coloring. A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four. That is at least three colors occur in a path and cycle of length four.
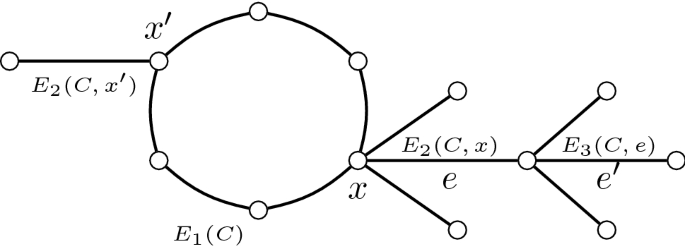Source: link.springer.com
Star edge-coloring of Halin graphs. For a graph G let the list star chromatic index of G ch. A star edge coloring of a graph is a proper edge coloring of such that every path and cycle of length four in uses at least three different colors. A star edge coloring of a graph G is a proper edge coloring of G such that every path and cycle of length four in G uses at least three different colors. A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four.
Source:
This is called a vertex coloring. The star chromatic number s G of Gis the least number of. In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color. A star k-edge-coloring is a proper k-edge-coloring such that every connected bicolored sub-graph is a path of length at most 3. Star edge-coloring of Halin graphs.
Source: wikiwand.com
In this paper we prove that 1 if G is a graph with Δ 4 then χ st G 14. Just like relation between concepts of traditional edge and vertex colorings a star coloring of a line graph is a star edge coloring of the original graph. A star coloring 1 4 5 of a graph Gis a proper vertex coloring in which every path on four vertices uses at least three distinct colors. In this article we establish tight upper bounds for trees and subcubic outerplanar graphs and derive an upper bound for outerplanar graphs. Dvořák Mohar and.
Source: sciencedirect.com
For a graph G let the list star chromatic index of G ch. We call a star edge coloring of Gwith kcolors a k-star edge coloring of G. Equivalently in a star coloring the induced subgraphs formed by the vertices of any two colors has connected components that are star graphs. The notion of the star edge coloring is intermediate. A star edge coloring of a graph is a proper edge coloring of such that every path and cycle of length four in uses at least three different colors.
This site is an open community for users to do submittion their favorite wallpapers on the internet, all images or pictures in this website are for personal wallpaper use only, it is stricly prohibited to use this wallpaper for commercial purposes, if you are the author and find this image is shared without your permission, please kindly raise a DMCA report to Us.
If you find this site good, please support us by sharing this posts to your preference social media accounts like Facebook, Instagram and so on or you can also save this blog page with the title star edge coloring of graphs by using Ctrl + D for devices a laptop with a Windows operating system or Command + D for laptops with an Apple operating system. If you use a smartphone, you can also use the drawer menu of the browser you are using. Whether it’s a Windows, Mac, iOS or Android operating system, you will still be able to bookmark this website.
