Your Star edge coloring of cactus graphs images are available. Star edge coloring of cactus graphs are a topic that is being searched for and liked by netizens today. You can Find and Download the Star edge coloring of cactus graphs files here. Get all free photos and vectors.
If you’re searching for star edge coloring of cactus graphs pictures information related to the star edge coloring of cactus graphs interest, you have visit the ideal blog. Our site always provides you with hints for refferencing the highest quality video and image content, please kindly search and locate more enlightening video content and images that match your interests.
Star Edge Coloring Of Cactus Graphs. A star edge coloring of a graph G is a proper edge coloring such that every connected bicolored subgraph is a path of length at most 3 the length of a path is the number of edges. The star chromatic index chi_stG of a graph G is the smallest integer k for which G has a proper k-edge coloring without bichromatic paths or cycles of length four. St G be the minimum k such that for any k-uniform list assignment L for the set of edges G has a star edge-coloring from L. In the following definitions and in the whole paper the term coloring will be used to define vertex coloring of graphs.
How To Make An Interesting Art Piece Using Tree Branches Ehow Plastic Canvas Crafts Plastic Canvas Patterns Plastic Canvas From pinterest.com
For example in Figure 41b the vertex w is incident to two edges. In this paper we prove that if H is a Halin graph. Note that for paths and cycles there exists a star edge coloring with at most 3 colors except for C. A star edge coloring of a graph G is a proper edge coloring such that every connected bicolored subgraph is a path of length at most 3 the length of a path is the number of edges. Like most cacti this plant is drought tolerant and doesnt need much maintenance. Graphs vertex coloring proper coloring star coloring.
One edge colored 6 then l1z6.
A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four. The exact size of your Christmas Cactus may vary depending on the size of the pot. The induced star arboricity isaG of a graph G is a new parameter introduced in 2019 by Axenovich et textital citeaxia2019 it is defined as the smallest number of induced star-forests covering the edges of G. A star edge coloring of a graph G is a proper edge coloring such that every connected bicolored subgraph is a path of length at most 3 the length of a path is the number of edges. In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color. For example in Figure 41b the vertex w is incident to two edges.
Source: link.springer.com
Colored 2and one edge colored 3 then l1w1 while the vertex z is incident with. Let GP 2 and HC n where n4 is even. Any local antimagic labeling induces a proper vertex coloring of G where the vertex u is assigned the color wu. And 2 if G is a bipartite graph with. The exact size of your Christmas Cactus may vary depending on the size of the pot.
Source: pinterest.com
One edge colored 6 then l1z6. Integer k such that G has a star k-edge-coloring. The list star chromatic index chstG is defined. Therefore f 6f. This is called a vertex coloringSimilarly an edge coloring assigns a color to each.
Source: semanticscholar.org
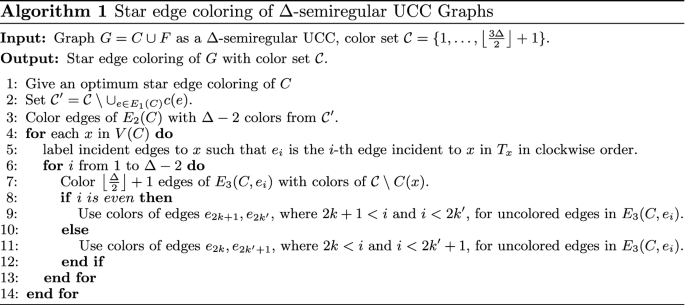
In this paper we prove that 1 if G is a graph with Δ 4 then χ st G 14. The star chromatic index of G is the smallest integer k for which G admits a star edge coloring with k colors. Star edge-coloring of Halin graphs. A star edge coloring of a graph G is a proper edge coloring of G such that no path or cycle of length 4 is bicolored. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively.
Source:
In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively. Now we prove the tightness of the bound. The plant itself costs between five and 15 dollars. Colored 2and one edge colored 3 then l1w1 while the vertex z is incident with. Star edge-coloring of Halin graphs.
Source: pinterest.com
In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color. In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color. It is an assignment of labels traditionally called colors to elements of a graph subject to certain constraints. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively. In the following definitions and in the whole paper the term coloring will be used to define vertex coloring of graphs.
Source: id.pinterest.com
A star edge coloring of a graph G is a proper edge coloring of G such that no path or cycle of length 4 is bicolored. This bound is tight. A star edge coloring of a graph G is a proper edge coloring such that every connected bicolored subgraph is a path of length at most 3 the length of a path is the number of edges. The star chromatic index chi_stG of a graph G is the smallest integer k for which G has a proper k-edge coloring without bichromatic paths or cycles of length four. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively.
Source: pinterest.com
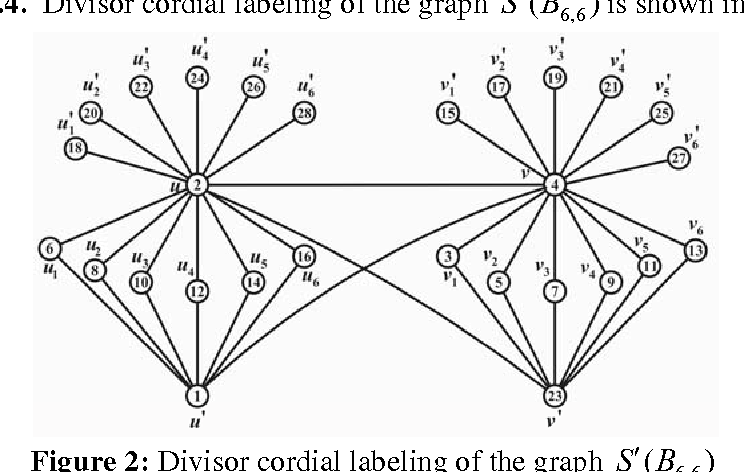
The star chromatic index χstG of a graph G is the smallest. A star edge-coloring of a graph G is a proper edge coloring such that every 2-colored connected subgraph of G is a path of length at most 3. This bound is tight. The star chromatic index of G denote Star Edge Coloring of Cactus Graphs. In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color.
Source: semanticscholar.org
Graphs vertex coloring proper coloring star coloring. A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four. The plant itself costs between five and 15 dollars. The star chromatic index chi_stG of a graph G is the smallest integer k for which G has a proper k-edge coloring without bichromatic paths or cycles of length four. Star edge-coloring of Halin graphs.
Source: link.springer.com
A star edge coloring of a graph G is a proper edge coloring such that every connected bicolored subgraph is a path of length at most 3 the length of a path is the number of edges. This bound is tight. In this paper we prove that if H is a Halin graph. The star chromatic index of G is the smallest integer k for which G admits a star edge coloring with k colors. In the following definitions and in the whole paper the term coloring will be used to define vertex coloring of graphs.
Source: pinterest.com
A star edge coloring of a graph is a proper edge coloring without bichromatic paths and cycles of length four. Let GP 2 and HC n where n4 is even. The star chromatic index of a graph is the smallest integer for which has a proper -edge coloring without bichromatic paths or cycles of length four. In this paper we prove that 1 if G is a graph with Δ 4 then χ st G 14. In this paper we prove that if H is a Halin graph.
Source: link.springer.com
For example in Figure 41b the vertex w is incident to two edges. Therefore f 6f. St G be the minimum k such that for any k-uniform list assignment L for the set of edges G has a star edge-coloring from L. For example in Figure 41b the vertex w is incident to two edges. The star chromatic index of a graph is the smallest integer for which has a proper -edge coloring without bichromatic paths or cycles of length four.
Source: link.springer.com
Colored 2and one edge colored 3 then l1w1 while the vertex z is incident with. A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four. The notion of the star edge coloring is intermediate between acyclic edge coloring when every bicolored subgraph is acyclic and strong edge coloring. The list star chromatic index chstG is defined. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively.
Source: pinterest.com
In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color. 1 Introduction All graphs considered here are undirected. A star edge-coloring of a graph G is a proper edge coloring such that every 2-colored connected subgraph of G is a path of length at most 3. Just like relation between concepts of traditional edge and vertex colorings a star coloring of a line graph is a star edge coloring of the original graph. The induced star arboricity isaG of a graph G is a new parameter introduced in 2019 by Axenovich et textital citeaxia2019 it is defined as the smallest number of induced star-forests covering the edges of G.
Source: pinterest.com
This shows that fis a star edge coloring of G H. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively. A star edge coloring of a graph G is a proper edge coloring such that every connected bicolored subgraph is a path of length at most 3 the length of a path is the number of edges. Coloring f1of G is given in Figure 41b and a 5-edge coloring f2of G is given in. A star edge-coloring of a graph G is a proper edge coloring such that every 2-colored connected subgraph of G is a path of length at most 3.
Source: pinterest.com
This is called a vertex coloringSimilarly an edge coloring assigns a color to each. A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four. It is an assignment of labels traditionally called colors to elements of a graph subject to certain constraints. The star chromatic index χ st G of a graph G is the smallest integer k for which G has a proper k -edge-coloring without bichromatic paths or cycles of length four. The list star chromatic index chstG is defined.
Source: researchgate.net
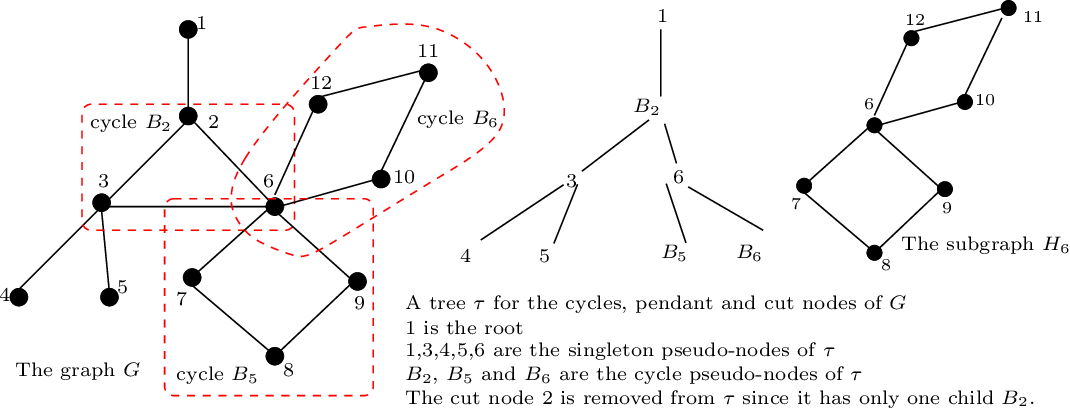
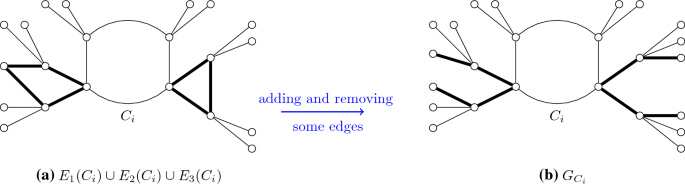
In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by P m C n P m W n P m H n P m G n respectively. The notion of the star edge coloring is intermediate between acyclic edge coloring when every bicolored subgraph is acyclic and strong edge coloring. In this paper we prove that if is a Halin graph with maximum degree then. In this article we establish tight upper bounds for trees and subcubic outerplanar graphs and derive an upper bound for outerplanar graphs. Consequently star edge colorings f c Hx and f c Hy are star compatible.
Source: pinterest.com
A star k-edge-coloring is a proper k-edge-coloring such that every connected bicolored sub-graph is a path of length at most 3. This bound is tight. Consequently star edge colorings f c Hx and f c Hy are star compatible. A star edge coloring of a graph G is a proper edge coloring without bichromatic paths and cycles of length four. For a graph G let the list star chromatic index of G ch.
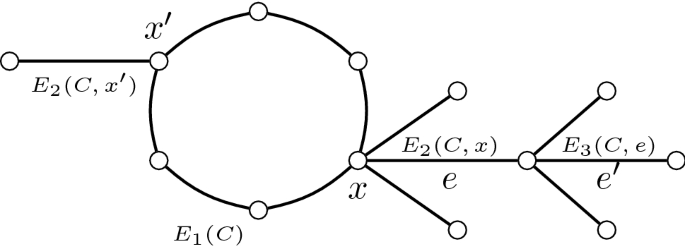Source: link.springer.com
An injective edge-coloring c of a graph G is an edge coloring such that if e_1 e_2 and e_3 are three consecutive edges in G they are consecutive if they form a path or a cycle of length three then e_1 and e_3 receive different colors. Therefore f 6f. Consequently star edge colorings f c Hx and f c Hy are star compatible. The plant itself costs between five and 15 dollars. In this paper we obtain the star edge chromatic number of the corona product of path with cycle path with wheel path with helm and path with gear graphs denoted by Pm Cn Pm Wn Pm Hn Pm Gn respectively.
This site is an open community for users to do submittion their favorite wallpapers on the internet, all images or pictures in this website are for personal wallpaper use only, it is stricly prohibited to use this wallpaper for commercial purposes, if you are the author and find this image is shared without your permission, please kindly raise a DMCA report to Us.
If you find this site value, please support us by sharing this posts to your favorite social media accounts like Facebook, Instagram and so on or you can also bookmark this blog page with the title star edge coloring of cactus graphs by using Ctrl + D for devices a laptop with a Windows operating system or Command + D for laptops with an Apple operating system. If you use a smartphone, you can also use the drawer menu of the browser you are using. Whether it’s a Windows, Mac, iOS or Android operating system, you will still be able to bookmark this website.
