Your Rainbow coloring graph theory images are ready. Rainbow coloring graph theory are a topic that is being searched for and liked by netizens today. You can Download the Rainbow coloring graph theory files here. Download all free photos and vectors.
If you’re searching for rainbow coloring graph theory pictures information related to the rainbow coloring graph theory interest, you have visit the ideal blog. Our website frequently gives you suggestions for seeking the maximum quality video and image content, please kindly surf and locate more informative video articles and graphics that fit your interests.
Rainbow Coloring Graph Theory. This average is the chromatic mean of v. The Fan graph denoted by F n can be constructed by joining n copies of cycle graph C. Rainbow faces in edge-colored plane graphs. A path is called a rainbow path if all of its edges have different colors.
Https Www Ijeat Org Wp Content Uploads Papers V9i2 B3725129219 Pdf From
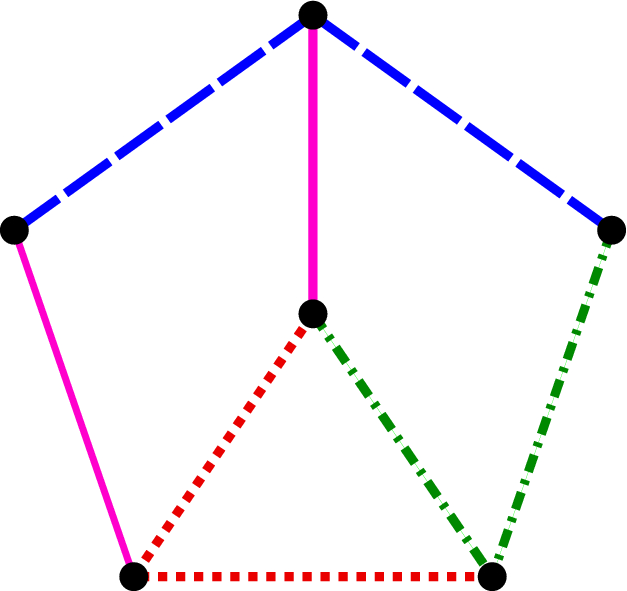
In graph theory rainbow coloring of graphs is an edge coloring technique of the graphs. Journal of Graph Theory 2009. A rainbow graph is a graph Γ that can be vertex-colored so that every color. For a nonempty graph F the. In graph theory a path in an edge-colored graph is said to be rainbow if no color repeats on it. On the other hand if all edges of F are colored differently then F is referred to as a rainbow F.
In graph theory rainbow coloring of graphs is an edge coloring technique of the graphs.
EG 1. If distinct vertices have distinct chromatic means then c is called a rainbow mean coloring. The rainbow connection number of a graph G denoted by rcG is the smallest number of k colors required. For a subgraph H of G let. If G is assigned such a coloring c then we say that G is a properly edge-colored graph or simply a properly colored graph. The adjacent edges may be colored the same colors.
Source: rainbowcoloringofgraphs.blogspot.com
Showed that computing the rainbow connection number of a general graph is NP-hard 2. The rainbow connection number of a graph G denoted by rcG is the smallest number of k colors required. Is represented once and only once in each neighborhood Γ 1v vVΓ. Such a coloring πVΓ Cwill be. We call an edge-coloring of a graph G a rainbow coloring if the edges of every quadrangle C4 in what follows of G are colored with distinct colors.
Source:
In fact even deciding whether rG 2 holds for a graph. An edge coloring of a graph is a function from its edge set to the set of natural numbers. On the other hand if all edges of F are colored differently then F is referred to as a rainbow F. K not necessarily proper. If distinct vertices have distinct chromatic means then c is called a rainbow mean coloring.
Source: researchgate.net
A subgraph H of G is called rainbow if its edges have distinct colors. The rainbow connection number and rainbow coloring have been studied from both the algorithmic and graph-theoretic points of view. Rainbow faces in edge-colored plane graphs. A graph is said to be rainbow colored if there is a rainbow path between each pair of its vertices. K not necessarily proper.
Source:
The map γ is called a rainbow coloring if any two vertices can be connected by a rainbow path. Is represented once and only once in each neighborhood Γ 1v vVΓ. Unlike in the case of ordinary colorings the goal is to maximize the number of used colors. This average is the chromatic mean of v. An edge colored graph G is rainbow connected if there exists a rainbow u v path for every two vertices u and v of G.
Source: researchgate.net
A path in an edge colored graph with no two edges sharing the same color is called a rainbow path. Let denote the color of the edge. A graph is said to be rainbow colored if there is a rainbow path between each pair of its vertices. The Fan graph denoted by F n can be constructed by joining n copies of cycle graph C. This average is the chromatic mean of v.
Source: sciencedirect.com
In graph theory a path in an edge-colored graph is said to be rainbow if no color repeats on it. A path in an edge colored graph with no two edges sharing the same color is called a rainbow path. Let G be a graph with an edge k-coloring γ. A subgraph H of G is called rainbow if its edges have distinct colors. A path in an edge colored graph is said to be a rainbow path if no two edges on the path have the same color.
Source: en.wikipedia.org
Is represented once and only once in each neighborhood Γ 1v vVΓ. A subgraph H of G is called rainbow if its edges have distinct colors. For a nonempty graph F the. A mean coloring of a connected graph G of order 3 or more is an edge coloring c of G with positive integers where the average of the colors of the edges incident with each vertex v of G is an integer. On the other hand if all edges of F are colored differently then F is referred to as a rainbow F.
Source: sciencedirect.com
In fact even deciding whether rG 2 holds for a graph. In fact even deciding whether rG 2 holds for a graph. Such a coloring πVΓ Cwill be. An edge-coloring of a loopless plane graph G is a facial rainbow edge-coloring if any two edges of G contained in the same facial path have distinct colors. This average is the chromatic mean of v.
Source: rainbowcoloringofgraphs.blogspot.com
Showed that computing the rainbow connection number of a general graph is NP-hard 2. The map γ is called a rainbow coloring if any two vertices can be connected by a rainbow path. EG 1. Basically in an edge-colored graph G that if there is a sub graph F of G all of whose edges are colored the same then F is referred to as a monochromatic F. Let rbG denote the minimum number of colors in a rainbow coloring of G.
Source: en.wikipedia.org
The adjacent edges may be colored the same colors. Let denote the color of the edge. Let G be a graph with an edge k-coloring γ. An edge coloring of a graph is a function from its edge set to the set of natural numbers. An edge colored graph G is rainbow connected if there exists a rainbow u v path for every two vertices u and v of G.
Source: link.springer.com
This average is the chromatic mean of v. A rainbow graph is a graph Γ that can be vertex-colored so that every color. The Fan graph denoted by F n can be constructed by joining n copies of cycle graph C. Such a coloring πVΓ Cwill be. We call an edge-coloring of a graph G a rainbow coloring if the edges of every quadrangle C4 in what follows of G are colored with distinct colors.
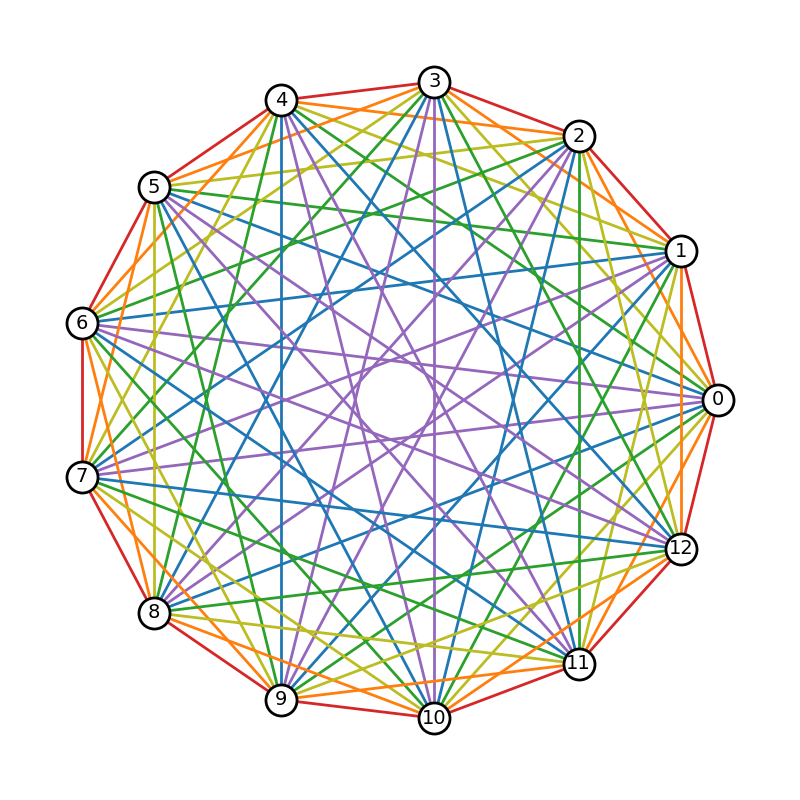Source: networkx.org
The Fan graph denoted by F n can be constructed by joining n copies of cycle graph C. We call an edge-coloring of a graph G a rainbow coloring if the edges of every quadrangle C4 in what follows of G are colored with distinct colors. K not necessarily proper. A subgraph H of G is called rainbow if its edges have distinct colors. In fact even deciding whether rG 2 holds for a graph.
Source: sciencedirect.com
In fact even deciding whether rG 2 holds for a graph. In graph theory rainbow coloring of graphs is an edge coloring technique of the graphs. Showed that computing the rainbow connection number of a general graph is NP-hard 2. An edge-coloring of a loopless plane graph G is a facial rainbow edge-coloring if any two edges of G contained in the same facial path have distinct colors. The adjacent edges may be colored the same colors.
Source: semanticscholar.org
The rainbow connection number of a graph G denoted by rcG is the smallest number of k colors required. A path in an edge colored graph with no two edges sharing the same color is called a rainbow path. A path in an edge colored graph is said to be a rainbow path if no two edges on the path have the same color. Rainbow faces in edge-colored plane graphs. Journal of Graph Theory 2009.
Source:
A graph is said to be rainbow colored if there is a rainbow path between each pair of its vertices. Rainbow faces in edge-colored plane graphs. A path in an edge colored graph with no two edges sharing the same color is called a rainbow path. For a subgraph H of G let. An edge colored graph G is rainbow connected if there exists a rainbow u v path for every two vertices u and v of G.
Source: wikiwand.com
The maximum number of colors that can be used in a non-rainbow coloring of a plane graph. Such a coloring πVΓ Cwill be. A subgraph H of G is called rainbow if its edges have distinct colors. Notice that rbG 1 if G has no quadrangles otherwise rbG 2 4. An edge-coloring of a loopless plane graph G is a facial rainbow edge-coloring if any two edges of G contained in the same facial path have distinct colors.
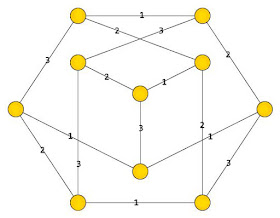Source: rainbowcoloringofgraphs.blogspot.com
For a nonempty graph F the. Notice that rbG 1 if G has no quadrangles otherwise rbG 2 4. In fact even deciding whether rG 2 holds for a graph. A path is called a rainbow path if all of its edges have different colors. The rainbow connection number of a graph G denoted by rcG is the smallest number of k colors required.
Source: sciencedirect.com
The facial rainbow edge-number of a graph G denoted mathrm erb G is the minimum number of colors that are necessary in any facial rainbow edge-coloring. EG 1. Is represented once and only once in each neighborhood Γ 1v vVΓ. For a nonempty graph F the. Notice that rbG 1 if G has no quadrangles otherwise rbG 2 4.
This site is an open community for users to share their favorite wallpapers on the internet, all images or pictures in this website are for personal wallpaper use only, it is stricly prohibited to use this wallpaper for commercial purposes, if you are the author and find this image is shared without your permission, please kindly raise a DMCA report to Us.
If you find this site good, please support us by sharing this posts to your own social media accounts like Facebook, Instagram and so on or you can also bookmark this blog page with the title rainbow coloring graph theory by using Ctrl + D for devices a laptop with a Windows operating system or Command + D for laptops with an Apple operating system. If you use a smartphone, you can also use the drawer menu of the browser you are using. Whether it’s a Windows, Mac, iOS or Android operating system, you will still be able to bookmark this website.
