Your Graph coloring local search images are available in this site. Graph coloring local search are a topic that is being searched for and liked by netizens now. You can Download the Graph coloring local search files here. Find and Download all royalty-free photos and vectors.
If you’re looking for graph coloring local search images information linked to the graph coloring local search interest, you have visit the ideal site. Our website always gives you hints for refferencing the maximum quality video and picture content, please kindly search and find more informative video content and graphics that fit your interests.
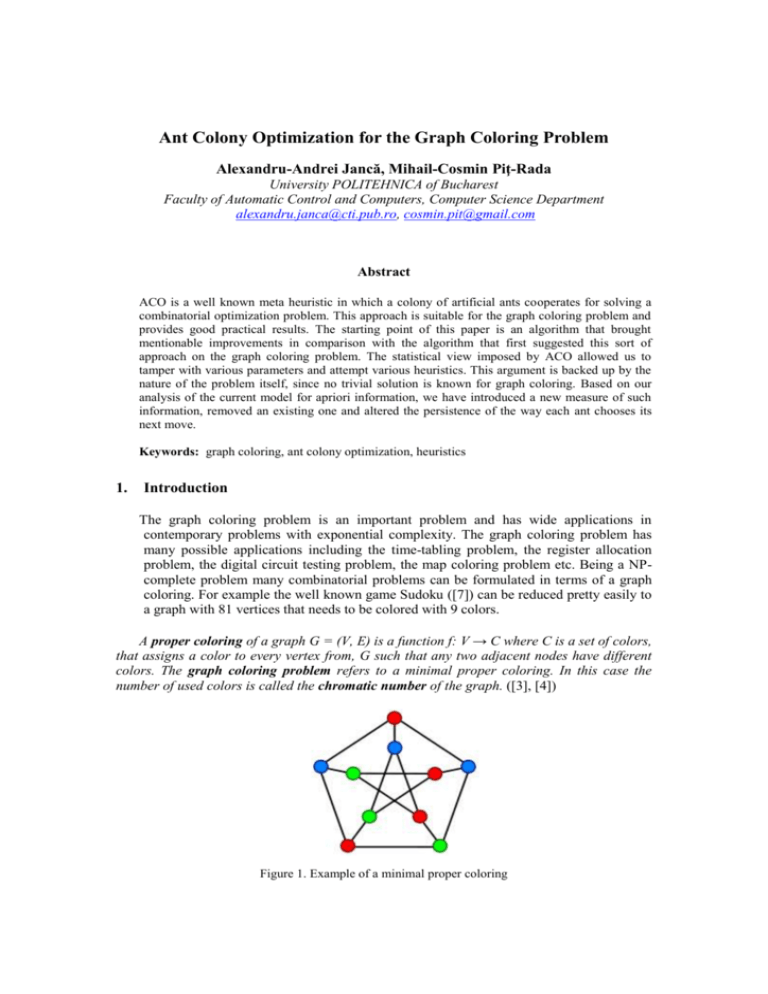
Graph Coloring Local Search. This is called a vertex coloring. For a given number of colors k one has to find a feasible coloring. This paper presents an improved probability learning based local search algorithm for the well-known graph coloring problem. It is an assignment of labels traditionally called colors to elements of a graph subject to certain constraints.
Pdf An Application Of Iterated Local Search To Graph Coloring Problem From researchgate.net
The algorithm combines an original crossover based on the notion of union of independent sets and a powerful local search operator tabu search. Graph coloring is a well known problem from graph theory that when attacking it with local search algorithms is typically treated as a series of constraint satisfaction problems. A hypothesis was experimentally. Tabucol is a local search algorithm to determine whether the vertices of an undi-rected graph can be colored with k colors such that no two vertices connected by an edge have the same color. GCP is very important because it has many applications. Graph coloring is a well known problem from graph theory that when solving it with local search algorithms is typically treated as a series of constraint satisfaction problems.
A case study on graph coloring.
Once such a coloring is found the number of colors is decreased and the local search starts again. Grouping problems aim to partition a set of items into multiple mutually disjoint subsets according to some specific criterion and constraints. In graph theory graph coloring is a special case of graph labeling. This paper presents a new genetic local search algorithm for the graph coloring problem. Once such a coloring is found the number of colors is decreased and the local search starts again. At the end of this video in a MAP region 1 is also Adjacent to region 4 Graph coloring problem using BacktrackingPATREON.
Source:
Similarly an edge coloring assigns a color to each edge so that no two adjacent edges are of the same color and a face coloring of a planar graph. Tabucol is a local search algorithm to determine whether the vertices of an undi-rected graph can be colored with k colors such that no two vertices connected by an edge have the same color. Similarly an edge coloring assigns a color to each edge so that no two adjacent edges share the same color and a face. This is called a vertex coloring. Graph coloring is a well known problem from graph theory that when attacking it with local search algorithms is typically treated as a series of constraint satisfaction problems.
Source: studylib.net
This is called a vertex coloring. Some of them are planning and scheduling problems 23 timetabling 4 map coloring 5 and many others. Given a graph G find xG and the corresponding coloring. We are interested in coloring graphs while using as few colors as possible. Similarly an edge coloring assigns a color to each edge so that no two adjacent edges are of the same color and a face coloring of a planar graph.
Source: sciencedirect.com
Formally a k-coloring of a graph is a function c. Tabucol is a local search algorithm to determine whether the vertices of an undi-rected graph can be colored with k colors such that no two vertices connected by an edge have the same color. Graph coloring is a well known problem from graph theory that when solving it with local search algorithms is typically treated as a series of constraint satisfaction problems. Journal of Graph Algorithms and Applications. Formally a k-coloring of a graph is a function c.
Source: github.com
The Graph Colouring Problem GCP is a well known NP-hard problem with many theoretical and practical applications. Yangming Zhou Jin-Kao Hao Béatrice Duval. We provide an extensive numerical comparison between this method and several other local search. Formally a k-coloring of a graph is a function c. A coloring of a graph is an assignment of one color to every vertex in a graph so that each edge attaches vertices of di erent colors.
Source: semanticscholar.org
V f1kgso that for all uv 2V cu 6 cv. A graph is k-colorable if it has a k-coloring. A hypothesis was experimentally. Tabucol is a local search algorithm to determine whether the vertices of an undi-rected graph can be colored with k colors such that no two vertices connected by an edge have the same color. This thesis presents an algorithm that solves the graph coloring problem with parallel Tabucol searches.
Source: sciencedirect.com
Tabucol is a local search algorithm to determine whether the vertices of an undi-rected graph can be colored with k colors such that no two vertices connected by an edge have the same color. Tabucol is a local search algorithm to determine whether the vertices of an undi-rected graph can be colored with k colors such that no two vertices connected by an edge have the same color. A starting coloring generation phase based on a probability matrix a heuristic coloring improvement phase and a learning based probability updating phase. We are interested in coloring graphs while using as few colors as possible. Grouping problems aim to partition a set of items into multiple mutually disjoint subsets according to some specific criterion and constraints.
Source: semanticscholar.org
Low-degree graph partitioning via local search with applications to constraint satisfaction max cut and coloring. Parameterized and Exact Computation Edward Lee12 1School of Computer Science and Engineering UNSW Sydney Australia 2Decision Sciences Group Data61 CSIRO Australia Semester 2 2017 E. Some of them are planning and scheduling problems 23 timetabling 4 map coloring 5 and many others. 3 Three search spaces for graph coloring Given a graph G VE with vertex set V and edge set E and given an integer k a k-coloring of G is a function c. GCP is a NP-hard problem 1.
Source: semanticscholar.org
A hypothesis was experimentally. A case study on graph coloring. Journal of Graph Algorithms and Applications. Formally a k-coloring of a graph is a function c. A hypothesis was experimentally.
Source: towardsdatascience.com
This thesis presents an algorithm that solves the graph coloring problem with parallel Tabucol searches. For a given number of colors k one has to find a feasible coloring. It is an assignment of labels traditionally called colors to elements of a graph subject to certain constraints. A coloring of a graph is an assignment of one color to every vertex in a graph so that each edge attaches vertices of di erent colors. In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices share the same color.
Source: researchgate.net
A hypothesis was experimentally. Similarly an edge coloring assigns a color to each edge so that no two adjacent edges share the same color and a face. Lee UNSW Randomized Algorithms Semester 2 2017 1 37. A case study on graph coloring Authors. Graph coloring is a well known problem from graph theory that when solving it with local search algorithms is typically treated as a series of constraint satisfaction problems.
Source: semanticscholar.org
For a given number of colors k one has to find a feasible coloring. 3 Three search spaces for graph coloring Given a graph G VE with vertex set V and edge set E and given an integer k a k-coloring of G is a function c. In graph theory graph coloring is a special case of graph labeling. The value cx of a vertex x is called the color of x. A case study on graph coloring.
Source: semanticscholar.org
Grouping problems aim to partition a set of items into multiple mutually disjoint subsets according to some specific criterion and constraints. Research Collection School Of Computing and Information Systems. A graph is k-colorable if it has a k-coloring. Once such a coloring is found the number of colors is decreased and the local search starts again. A case study on graph coloring.
Source: mdpi.com
Once such a coloring is found the number of colors is decreased and the local search starts again. Graph coloring is a well known problem from graph theory that when solving it with local search algorithms is typically treated as a series of constraint satisfaction problems. GCP is very important because it has many applications. Once such a coloring is found the number of colors is decreased and the local search starts again. Some of them are planning and scheduling problems 23 timetabling 4 map coloring 5 and many others.
Source: slideplayer.com
Grouping problems aim to partition a set of items into multiple mutually disjoint subsets according to some specific criterion and constraints. A starting coloring generation phase based on a probability matrix a heuristic coloring improvement phase and a learning based probability updating phase. Once such a coloring is found the number of colors is decreased and the local search starts again. Given a graph G find xG and the corresponding coloring. Research Collection School Of Computing and Information Systems.
Source: in.pinterest.com
The Graph k-Colorability Problem GCP can be stated as follows. This paper presents a new genetic local search algorithm for the graph coloring problem. This is called a vertex coloring. This thesis presents an algorithm that solves the graph coloring problem with parallel Tabucol searches. A hypothesis was experimentally.
Source: slideplayer.com
The value cx of a vertex x is called the color of x. Graph coloring is a well known problem from graph theory that when attacking it with local search algorithms is typically treated as a series of constraint satisfaction problems. Tabucol is a local search algorithm to determine whether the vertices of an undi-rected graph can be colored with k colors such that no two vertices connected by an edge have the same color. In its simplest form it is a way of coloring the vertices of a graph such that no two adjacent vertices are of the same color. It is an assignment of labels traditionally called colors to elements of a graph subject to certain constraints.
Source: researchgate.net
GCP is very important because it has many applications. V f1kgso that for all uv 2V cu 6 cv. For a given number of colors k one has to find a feasible coloring. A case study on graph coloring Authors. In this paper we introduce a new local search algorithm based on a very large scale neighbourhood.
Source: researchgate.net
Once such a coloring is found the number of colors is decreased and the local search starts again. Grouping problems aim to partition a set of items into multiple mutually disjoint subsets according to some specific criterion and constraints. Graph coloring is a well known problem from graph theory that when solving it with local search algorithms is typically treated as a series of constraint satisfaction problems. The algorithm iterates through three distinct phases. A hypothesis was experimentally.
This site is an open community for users to submit their favorite wallpapers on the internet, all images or pictures in this website are for personal wallpaper use only, it is stricly prohibited to use this wallpaper for commercial purposes, if you are the author and find this image is shared without your permission, please kindly raise a DMCA report to Us.
If you find this site value, please support us by sharing this posts to your favorite social media accounts like Facebook, Instagram and so on or you can also save this blog page with the title graph coloring local search by using Ctrl + D for devices a laptop with a Windows operating system or Command + D for laptops with an Apple operating system. If you use a smartphone, you can also use the drawer menu of the browser you are using. Whether it’s a Windows, Mac, iOS or Android operating system, you will still be able to bookmark this website.
